Tutorials: Difference between revisions
mNo edit summary |
No edit summary |
||
| Line 1: | Line 1: | ||
__NOTOC__ | __NOTOC__ | ||
== [[Stochastic dynamics in finite populations]] == | |||
[[Image:Stochastic dynamics - noise term Cxx, no mutations.png|left|160x160px]] | |||
Tutorial on the stochastic dynamics arising through demographic noise and mutations in finite populations of size \(N\). Comparisons of the deterministic replicator dynamics in the limit of infinite population sizes \(N\to\infty\) to the stochastic dynamics generated by stochastic differential equations, which are derived from a microscopic description of elementary changes in the population, as well as to results from individual based simulations. | |||
<div class="footnote" style="margin-left:160px;font-size:smaller"> | |||
#Traulsen, A., Claussen, J. C. & Hauert, C. (2012) ''Phys. Rev. E'' in print. | |||
#Traulsen, A., Claussen, J. C. & Hauert, C. (2006) ''Phys. Rev. E'' '''74''' 011901 [http://dx.doi.org/10.1103/PhysRevE.74.011901 doi: 10.1103/PhysRevE.74.011901]. | |||
#Traulsen, A., Claussen, J. C. & Hauert, C. (2005) ''Phys. Rev. Lett.'' '''95''' 238701 [http://dx.doi.org/10.1103/PhysRevLett.95.238701 doi: 10.1103/PhysRevLett.95.238701]. | |||
</div> | |||
{{-}} | |||
== [[Evolutionary Games and Population Dynamics]] == | |||
[[Image:Evolutionary games and population dynamics.gif|left|160x160px|link=Evolutionary Games and Population Dynamics]] | |||
Tutorial on frequency dependent selection in populations of varying size. The classic replicator dynamics assumes constant (infinite) population sizes and thus neglects the ecology of the population. Linking ecological dynamics and evolutionary games generates fascinating and rich dynamical behavior. Most importantly, however, this reveals a new mechanism for maintaining cooperation through negative feedback between population densities and the size of interaction groups. | |||
<div class="footnote" style="margin-left:160px;font-size:smaller"> | |||
# Wakano, J. Y. & Hauert, Ch. (2011) J. theor. Biol. 268 30-38 [http://dx.doi.org/10.1016/j.jtbi.2010.09.036 doi: 10.1016/j.jtbi.2010.09.036]. | |||
# Wakano, J. Y., Nowak, M. A. & Hauert, Ch. (2009) Proc. Natl. Acad. Sci. USA 106 7910-7914 [http://dx.doi.org/10.1073/pnas.0812644106 doi: 10.1073/pnas.0812644106]. | |||
# Hauert, Ch., Wakano, J. Y. & Doebeli, M. (2008) Theor. Pop. Biol. '''73''', 257-263 [http://dx.doi.org/10.1016/j.tpb.2007.11.007 doi:10.1016/j.tpb.2007.11.007]. | |||
# Hauert, C., Holmes, M. & Doebeli, M. (2006) Proc. R. Soc. Lond. B '''273''', 2565-2570 [http://dx.doi.org/10.1098/rspb.2006.3600 doi: 10.1098/rspb.2006.3600]. | |||
</div> | |||
{{-}} | |||
== [[Origin of Cooperators and Defectors]] == | |||
[[Image:Origin of cooperators and defectors.gif|left|160x160px|link=Origin of Cooperators and Defectors]] | |||
Tutorial on the gradual evolution of distinct cooperative and defective behavioral patterns through evolutionary branching into separate trait groups characterized by high and low cooperative investments. This is based on a model that extends the classical Snowdrift game to continuously varying degrees of cooperation. Apart from evolutionary branching, this model exhibits rich dynamics that can be easily explored using this interactive tutorial. | |||
<div class="footnote" style="margin-left:160px;font-size:smaller"> | |||
# Killingback, T., Doebeli, M. & Hauert, Ch. (2010) Biological Theory 5, 3-6 [http://dx.doi.org/10.1162/BIOT_a_00019 doi: 10.1162/BIOT_a_00019]. | |||
# Doebeli, M., Hauert, C. & Killingback, T. (2004) Science '''306''', 859-862 [http://dx.doi.org/10.1126/science.1101456 doi: 10.1126/science.1101456]. | |||
</div> | |||
{{-}} | |||
== [[2×2 Games]] == | |||
[[Image:Cover IJBC 2002.12.png|left|160x160px|link=2×2 Games]] | |||
Tutorial on 2×2 games in populations with different structures. 2×2 games describe a rich set of pairwise interactions among individuals. The most prominent game is certainly the Prisoner's Dilemma which has become the paradigm to discuss the emergence of cooperative behavior. If players are arranged on regular lattices, many of these games produce fascinating spatio-temporal patterns. This tutorial provides a hands-on experience of this dynamical world. | |||
<div class="footnote" style="margin-left:126px;font-size:smaller"> | |||
# Hauert, C. (2002) Int. J. of Bifurcation & Chaos '''12''' 1531-1548 [http://dx.doi.org/10.1142/S0218127402005273 doi: 10.1142/S0218127402005273]. | |||
# Hauert, C. (2001) Proc. R. Soc. Lond. B 268, 761-769 [http://dx.doi.org/10.1098/rspb.2000.1424 doi: 10.1098/rspb.2000.1424]. | |||
</div> | |||
{{-}} | |||
<!-- | |||
== [[Introduction]] == | == [[Introduction]] == | ||
[[Image:Founding fathers of Game Theory.gif|left|border|160x160px]] | [[Image:Founding fathers of Game Theory.gif|left|border|160x160px]] | ||
Quick introduction covering the basic ideas of evolutionary game theory illustrated with many examples. This section briefly introduces classic games such as the Prisoner's Dilemma, the Hawk-Dove- or Snowdrift game and the Rock-Scissors-Paper game. It is intended as an overview of the many features and capabilities of the interactive ''EvoLudo'' simulator. | Quick introduction covering the basic ideas of evolutionary game theory illustrated with many examples. This section briefly introduces classic games such as the Prisoner's Dilemma, the Hawk-Dove- or Snowdrift game and the Rock-Scissors-Paper game. It is intended as an overview of the many features and capabilities of the interactive ''EvoLudo'' simulator. | ||
{{-}} | {{-}} | ||
--> | |||
<!-- | |||
== [[Via freedom to coercion: the emergence of costly punishment]] == | == [[Via freedom to coercion: the emergence of costly punishment]] == | ||
[[Image:Via freedom to coercion.gif|left|160x160px]] | [[Image:Via freedom to coercion.gif|left|160x160px]] | ||
| Line 12: | Line 61: | ||
<div class="footnote"> Hauert, C., Traulsen, A., Brandt, H., Nowak, M. A. & Sigmund, K. (2007) ''Via freedom to coercion: the emergence of costly punishment,'' ''Science'' '''316''', 1905-1907. </div> | <div class="footnote"> Hauert, C., Traulsen, A., Brandt, H., Nowak, M. A. & Sigmund, K. (2007) ''Via freedom to coercion: the emergence of costly punishment,'' ''Science'' '''316''', 1905-1907. </div> | ||
{{-}} | {{-}} | ||
--> | |||
<!-- | |||
== [[Synergy & discounting of cooperation in social dilemmas]] == | == [[Synergy & discounting of cooperation in social dilemmas]] == | ||
[[Image:Synergy & discounting in social dilemmas.gif|left|160x160px]] | [[Image:Synergy & discounting in social dilemmas.gif|left|160x160px]] | ||
| Line 26: | Line 69: | ||
<div class="footnote"> Hauert, C., Michor, F., Nowak, M. & Doebeli, M. (2006) J. theor. Biol. '''239''', 195-202.<br /> Hauert, C. (2006) J. theor. Biol. '''240''', 627-636. </div> | <div class="footnote"> Hauert, C., Michor, F., Nowak, M. & Doebeli, M. (2006) J. theor. Biol. '''239''', 195-202.<br /> Hauert, C. (2006) J. theor. Biol. '''240''', 627-636. </div> | ||
{{-}} | {{-}} | ||
--> | |||
<!-- | |||
< | |||
== [[Evolutionary dynamics on graphs]] == | == [[Evolutionary dynamics on graphs]] == | ||
[[Image:Evolutionary dynamics on graphs.gif|left|160x160px]] | [[Image:Evolutionary dynamics on graphs.gif|left|160x160px]] | ||
| Line 40: | Line 77: | ||
<div class="footnote"> Lieberman, E., Hauert, C. & Nowak, M. (2005) Nature '''433''', 312-316.<br /> Ohtsuki, H., Hauert, C., Lieberman, E. & Nowak, M. (2006) Nature '''441''', 502-505. </div> | <div class="footnote"> Lieberman, E., Hauert, C. & Nowak, M. (2005) Nature '''433''', 312-316.<br /> Ohtsuki, H., Hauert, C., Lieberman, E. & Nowak, M. (2006) Nature '''441''', 502-505. </div> | ||
{{-}} | {{-}} | ||
--> | |||
<!-- | |||
== [[Cooperation in structured populations]] == | == [[Cooperation in structured populations]] == | ||
[[Image:Cooperation in structured populations.gif|left|border|160x160px]] | [[Image:Cooperation in structured populations.gif|left|border|160x160px]] | ||
| Line 47: | Line 85: | ||
<div class="footnote"> Hauert, C. & Doebeli, M. (2004) Nature '''428''', 643-646. </div> | <div class="footnote"> Hauert, C. & Doebeli, M. (2004) Nature '''428''', 643-646. </div> | ||
{{-}} | {{-}} | ||
--> | |||
<!-- | |||
== [[Voluntary Public Goods Games]] == | == [[Voluntary Public Goods Games]] == | ||
[[Image:Voluntary Public Goods Games.gif|left|border|160x160px]] | [[Image:Voluntary Public Goods Games.gif|left|border|160x160px]] | ||
| Line 54: | Line 93: | ||
<div class="footnote"> Hauert, C., De Monte, S., Hofbauer, J. & Sigmund, K. (2002) Science '''296''' 1129-1132.<br /> Hauert, C., De Monte, S., Hofbauer, J. & Sigmund, K. (2002) J. theor. Biol. '''218''' 187-194. </div> | <div class="footnote"> Hauert, C., De Monte, S., Hofbauer, J. & Sigmund, K. (2002) Science '''296''' 1129-1132.<br /> Hauert, C., De Monte, S., Hofbauer, J. & Sigmund, K. (2002) J. theor. Biol. '''218''' 187-194. </div> | ||
{{-}} | {{-}} | ||
--> | |||
<!-- | |||
== [[Public goods games]] == | == [[Public goods games]] == | ||
[[Image:Public goods games.gif|left|border|160x160px]] | [[Image:Public goods games.gif|left|border|160x160px]] | ||
| Line 61: | Line 101: | ||
<div class="footnote"> Szabó, G. & Hauert, C. (2002) Phys. Rev. Lett. '''89''' (11) 118101. </div> | <div class="footnote"> Szabó, G. & Hauert, C. (2002) Phys. Rev. Lett. '''89''' (11) 118101. </div> | ||
{{-}} | {{-}} | ||
--> | |||
<!-- | |||
== [[Reward, punishment and reputation]] == | == [[Reward, punishment and reputation]] == | ||
[[Image:Reward, punishment & reputation.gif|left|border|160x160px]] | [[Image:Reward, punishment & reputation.gif|left|border|160x160px]] | ||
| Line 68: | Line 109: | ||
<div class="footnote"> Sigmund, K., Hauert, C. & Nowak, M. (2001) Proc. Natl Acad. Sci. USA '''98''', 10757-10762.<br /> Brandt, H., Hauert, C. & Sigmund, K. (2003) Proc. R. Soc. Lond. B '''270''', 1099-1104. </div> | <div class="footnote"> Sigmund, K., Hauert, C. & Nowak, M. (2001) Proc. Natl Acad. Sci. USA '''98''', 10757-10762.<br /> Brandt, H., Hauert, C. & Sigmund, K. (2003) Proc. R. Soc. Lond. B '''270''', 1099-1104. </div> | ||
{{-}} | {{-}} | ||
--> | |||
Revision as of 16:31, 21 March 2012
Stochastic dynamics in finite populations

Tutorial on the stochastic dynamics arising through demographic noise and mutations in finite populations of size \(N\). Comparisons of the deterministic replicator dynamics in the limit of infinite population sizes \(N\to\infty\) to the stochastic dynamics generated by stochastic differential equations, which are derived from a microscopic description of elementary changes in the population, as well as to results from individual based simulations.
- Traulsen, A., Claussen, J. C. & Hauert, C. (2012) Phys. Rev. E in print.
- Traulsen, A., Claussen, J. C. & Hauert, C. (2006) Phys. Rev. E 74 011901 doi: 10.1103/PhysRevE.74.011901.
- Traulsen, A., Claussen, J. C. & Hauert, C. (2005) Phys. Rev. Lett. 95 238701 doi: 10.1103/PhysRevLett.95.238701.
Evolutionary Games and Population Dynamics
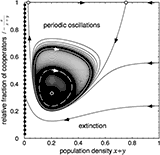
Tutorial on frequency dependent selection in populations of varying size. The classic replicator dynamics assumes constant (infinite) population sizes and thus neglects the ecology of the population. Linking ecological dynamics and evolutionary games generates fascinating and rich dynamical behavior. Most importantly, however, this reveals a new mechanism for maintaining cooperation through negative feedback between population densities and the size of interaction groups.
- Wakano, J. Y. & Hauert, Ch. (2011) J. theor. Biol. 268 30-38 doi: 10.1016/j.jtbi.2010.09.036.
- Wakano, J. Y., Nowak, M. A. & Hauert, Ch. (2009) Proc. Natl. Acad. Sci. USA 106 7910-7914 doi: 10.1073/pnas.0812644106.
- Hauert, Ch., Wakano, J. Y. & Doebeli, M. (2008) Theor. Pop. Biol. 73, 257-263 doi:10.1016/j.tpb.2007.11.007.
- Hauert, C., Holmes, M. & Doebeli, M. (2006) Proc. R. Soc. Lond. B 273, 2565-2570 doi: 10.1098/rspb.2006.3600.
Origin of Cooperators and Defectors

Tutorial on the gradual evolution of distinct cooperative and defective behavioral patterns through evolutionary branching into separate trait groups characterized by high and low cooperative investments. This is based on a model that extends the classical Snowdrift game to continuously varying degrees of cooperation. Apart from evolutionary branching, this model exhibits rich dynamics that can be easily explored using this interactive tutorial.
- Killingback, T., Doebeli, M. & Hauert, Ch. (2010) Biological Theory 5, 3-6 doi: 10.1162/BIOT_a_00019.
- Doebeli, M., Hauert, C. & Killingback, T. (2004) Science 306, 859-862 doi: 10.1126/science.1101456.
2×2 Games

Tutorial on 2×2 games in populations with different structures. 2×2 games describe a rich set of pairwise interactions among individuals. The most prominent game is certainly the Prisoner's Dilemma which has become the paradigm to discuss the emergence of cooperative behavior. If players are arranged on regular lattices, many of these games produce fascinating spatio-temporal patterns. This tutorial provides a hands-on experience of this dynamical world.
- Hauert, C. (2002) Int. J. of Bifurcation & Chaos 12 1531-1548 doi: 10.1142/S0218127402005273.
- Hauert, C. (2001) Proc. R. Soc. Lond. B 268, 761-769 doi: 10.1098/rspb.2000.1424.
