Spatial games: interaction and competition neighbourhoods
The donation game is a particularly convenient instance of a social dilemma with a single parameter given by the cost-to-benefit ratio of cooperation, \(r\). In spatial settings limited local interactions and clustering are capable of supporting cooperation by reducing exploitation from defectors. Traditionally the interaction and competition neighbourhoods are identical. Here we discuss intriguing differences in the dynamics that arise when separating the neighbourhoods. On the square lattice separate interaction and competition neighbourhoods can be easily realized by considering nearest neighbour interactions and second nearest neighbour competition. Incidentally, the number of first and second neighbours is the same. For competition this results in two equivalent disjoint sub-lattices and hence is ideally suited to investigate effects of separating interactions and competition. The extinction of cooperators for higher cost-to-benefit ratios \(r\) remains the same in both scenarios and exhibits a critical phase transition with characteristics of directed percolation. However, for smaller \(r\) significant differences to the traditional setup with overlapping neighbourhoods arise: spiteful behaviour is suppressed and, even more intriguingly, distinct levels of cooperation can emerge in the two sub-lattices through spontaneous symmetry breaking. The symmetry breaking resembles the sub-lattice ordering occurring in the anti-ferromagnetic Ising model. Within the twofold degenerated phases, decreasing the cost-to-benefit ratio induces extremely large fluctuations (bursts) in the frequencies of cooperation. These bursts eventually drive the system into one of the absorbing states: occasionally homogeneous defection in both sub-lattices but usually only in one and homogeneous cooperation in the other, achieving perfect asymmetry. This is in stark contrast to the traditional case where cooperation keeps increasing for decreasing \(r\) and hence more benign conditions.
The donation game
Spatial populations
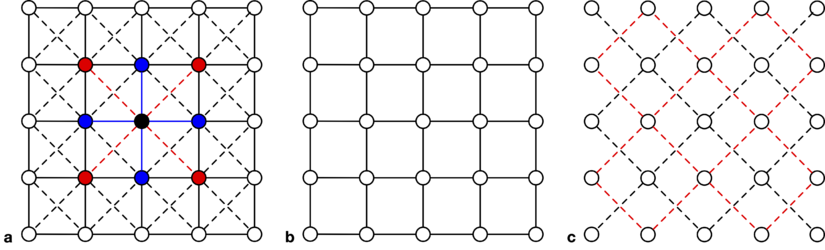Figure 1: a Schematic depiction of the disjoint interaction (\(\bullet\)) and competition (\(\bullet\)) neighbourhoods of the focal individual (\(\bullet\)) on a square lattice. b The interaction network is the standard 2D square lattice with degree \(k = 4\) and includes all members of the population. c In contrast, the reproduction (or imitation) network connects the \(l = 4\) second-nearest neighbours along the diagonals. This results in two disjoint sub-lattices (black and red dashed lines, respectively) of equal sizes, provided that \(L\) is even on an \(L\times L\) lattice.
Overlapping neighbourhoods
Dynamical domains
- \(r>r_C\): defection dominates. For high costs or low benefits, spatial correlations are insufficient to support cooperation. The outcome is the same as in well-mixed populations, see \eqref{eq:remut}.
- \(r_D<r<r_C\): cooperators and defectors co-exist. Note that \(r_D\approx XXX<0\). Strictly speaking the donation game only applies for \(r>0\) and turns into a harmony game for \(r<0\). This implies that for \(r<0\) defection becomes an act of spite, which is costly to the actor but harms others more.
- \(r<r_D\): cooperation dominates. The costs for spiteful behaviour become too high and defectors disappear.
The three dynamical regimes are separated by critical phase transitions that fall into the universality class of directed percolation.
Spiteful behaviour in spatial donation game
Cooperators and defectors co-exist through spiteful behaviour.
Disjoint interaction and competition neighbourhoods
Dynamical domains
- \(r>r_C\): defection dominates. XXX.
- \(r_S<r<r_C\): cooperators and defectors co-exist, symmetric phase. Frequencies of cooperation are identical in both sub-lattices.
- \(r_A<r<r_S\): cooperators and defectors co-exist, asymmetric phase. Frequencies of cooperation are almost complementary in the two sub-lattices.
- \(r<r_A\): absorbing states. XXX
The four dynamical regimes are separated by different kinds of critical phase transitions: the first, at \(r_C\approx XXX\) again falls into the universality class of directed percolation; at \(r_S\approx XXX\) the spontaneous symmetry breaking follows the Ising-type universality class; and finally at \(r_A\) bursts of defection drive the population into absorbing states. XXX
Disjoint competition in spatial donation game, symmetric phase
Cooperators and defectors co-exist at equal frequencies in both sub-lattices.
Disjoint competition in spatial donation game, asymmetric phase
Cooperators and defectors co-exist at complimentary frequencies in both sub-lattices.