2×2 Games/Well-mixed populations: Difference between revisions
No edit summary |
mNo edit summary |
||
| Line 1: | Line 1: | ||
{{InCharge|author1=Christoph Hauert}} | {{InCharge|author1=Christoph Hauert}} | ||
__NOTOC__ | __NOTOC__ | ||
[[Image:Well-mixed 2x2 Games.png|thumb|300px|Four basic evolutionary scenarios of 2×2 Games in the | [[Image:Well-mixed 2x2 Games.png|thumb|300px|Four basic evolutionary scenarios of 2×2 Games in the | ||
In well-mixed populations the equilibrium fractions of cooperators and defectors are easily calculated using the replicator equation. If | In well-mixed populations the equilibrium fractions of cooperators and defectors are easily calculated using the replicator equation. If | ||
Revision as of 22:14, 22 March 2012
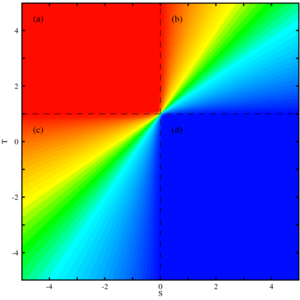
In well-mixed populations the equilibrium fractions of cooperators and defectors are easily calculated using the replicator equation. If
\[\dot x = \frac{dx}{dt} = x(1-x)(P_A-P_B)\]
where
\[x_3 = \frac{P-S}{R-S-T+P}.\]
The replicator equation allows to shift and normalize the payoffs without affecting the dynamics because the performance of cooperators and defectors only depends on the relative payoffs, i.e. on payoff differences. For this reason we can set
Basic evolutionary scenarios
All of the following examples and suggestions are meant as inspirations for further experimenting with the EvoLudo simulator. Each of following examples starts a lab that demonstrates the particular dynamical scenario. By modifying the parameters the dynamics can be further explored.
| Color code: | Type A | Type B |
|---|

Type A dominates
For
If

Type B dominates
Conversely, for
If

Coexistence
For
This situation corresponds to the Snowdrift game, Chicken or Hawk-Dove game used to model cooperation and competition in biology (for further details see the tutorial on Cooperation in structured populations).
Bistability
Finally, for
This situation represent a coordination game often referred to as a Staghunt game.
